Electrical Activity

A key aspect of the heart modeling is the study of electrical activity, that triggers the heart contraction.

A simple model considered is the Eikonal equation which provides the activation times. In view of the electro-mechanical coupling more sophisticated models have been used, in particular the Monodomain and the Bidomain models, which allow to compute also the transmembrane potential.

The research focused on the algebraic solution of the Bidomain problem, for example by developing  and analyzing a new preconditioner based on the solution of the Monodomain equation, or by introducing an hybrid model (Hybridomain model) based on an a posteriori estimator. Also new time discretization schemes to solve the ODE system describing the ionic currents have been proposed.

Another topic concerned the generation of the Purkinje fibers that spread the electrical signal toward the ventricular myocardium. In this respect a new algorithm for the generation of a patient-specific Purkinje network has been developed.  This methodology has been applied to real cases using patient-specific clinical data on the activation times acquired by clinicians with the NavX system.

Several applications to pathological cases has been considered, such as arrhythmias, Wolff-Parkinson-White syndrome, left bundle branch block, myocardial ischemia.

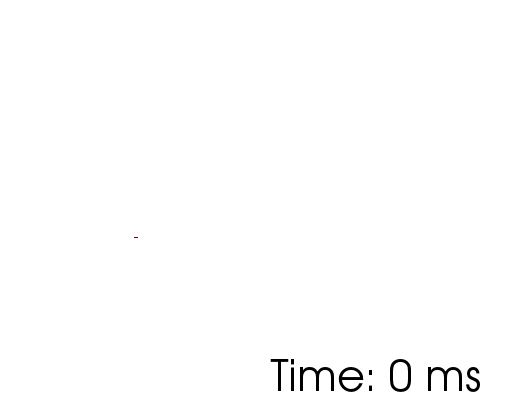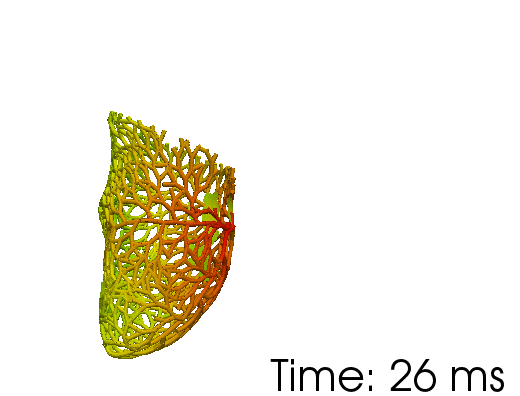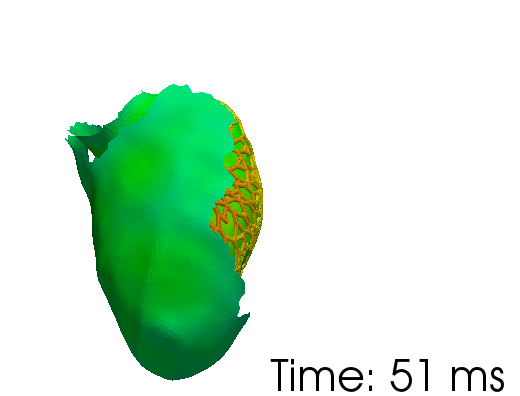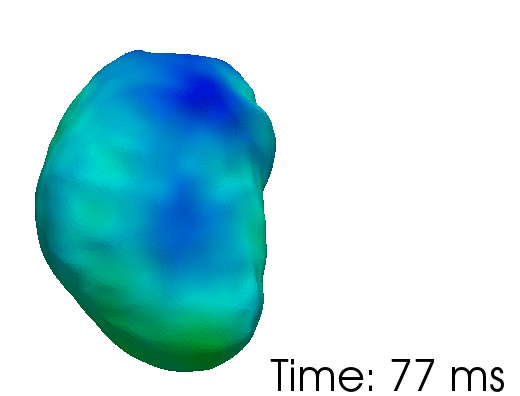
Publications:

1. Gerardo-Giorda L., Mirabella L., Nobile F., Perego M., Veneziani A., A model-based block-triangular preconditioner for the bidomain system in electrocardiology, J. Comput. Physics, 228(10), pp. 3625-3639, 2009.
2. Perego M., Veneziani A., An efficient generalization of the Rush-Larsen method for solving electro-physiology membrane equations, Electr. Trans. on Numer. Anal., 35, pp. 234-256, 2009.
3. Clayton R.H., Bernus O., Cherry E.M., Dierckx H., Fenton F.H., Mirabella L., Panfilov A.V., Sachse F.B., Seemann G., Zhang H., Models of cardiac tissue electrophysiology: Progress, challenges and open questions, Prog. Biophys. Mol. Biol., 104(1-3), pp. 22-48, 2011.
4. Mirabella L., Nobile F., Veneziani A., An a posteriori error estimator for model adaptivity in electrocardiology, Comp. Meth. Appl. Meth. Eng., 200(37-40), pp. 2727-2737, 2011.
5. Vergara C., Palamara S., Catanzariti D., Pangrazzi C., Nobile F., Centonze M., Faggiano E., Maines M., Quarteroni A., Vergara G., Patient-specific generation of the purkinje network driven by clinical measurements of a normal propagation, Med. Biol. Eng. Comput., 52(10), pp. 813-826, 2014.
6. Palamara S., Vergara C., Catanzariti D., Faggiano E., Pangrazzi C., Centonze M., Quarteroni A.,  Computational generation of the Purkinje network driven by clinical measurements: The case of pathological propagations, Int. J. Numer. Meth. Biomed. Eng. ,30(12), pp. 1558-77, 2014.
7. Palamara S., Vergara C., Faggiano E., Nobile F.,  An effective algorithm for the generation of patient-specific Purkinje networks in computational electrocardiology, J. Comput. Physics, (283),  pp. 495-517, 2015.
8. Lange M., Palamara S., Lassila T., Vergara C., Quarteroni A., Frangi A.F., Efficient Numerical Schemes for Computing Cardiac Electrical Activation over Realistic Purkinje Networks: Method and Verification, in “Functional Imaging and Modeling of the Heart”, Proceedings of the 8th International Conference, FIMH 2015, Springer, pp. 430-438, 2015
9. M. Dupraz, S. Filippi, A. Gizzi, A. Quarteroni and R. Ruiz-Baier. Finite element and finite volume-element simulation of pseudo-ECGs and cardiac alternans, in Mathematical Methods in the Applied Sciences, vol. 38, num. 6, p. 1046-1058, 2015.
10. Vergara C., Lange M., Palamara S., Lassila T., Frangi A.F., Quarteroni A., A coupled 3D-1D numerical monodomain solver for cardiac electrical activation in the myocardium with detailed Purkinje network. Journal of Computational Physics, 308, pp. 218–238, 2016
11. Lange M., Palamara S., Lassila T., Vergara C., Quarteroni A., Frangi A.F., Improved hybrid/GPU algorithm for solving cardiac electrophysiology problems on Purkinje networks.. Int. J. Numer. Meth. Biomed. Eng.. DOI: 10.1002/cnm.2835

Thesis:

1. N. Papucci – Comparaison de Méthodes d intégration en temps en électrocardiologie – BSc in Mathematical Engineering – Politecnico di Milano – Advisor: F. Nobile – A.A ’08-’09
2. S. Pezzuto – Adattività spazio-tempo per sistemi di reazione-diffusione non lineari con applicazioni all’elettrocardiologia – MSc in Mathematical Engineering – Politecnico di Milano – Advisor: F. Nobile – A.A. ’08-’09
3. L. Mirabella – Numerical methods for cardiovascular problems: computational electrocardiology and fluid dynamics in moving domains Ph.D. thesis in Mthematical Engineeing – MOX, Dipartimento di Matematica, Politecnico di Milano – 2010 -Advisor: A. Veneziani
4. M. Perego – Mathematical and Numerical Models for Focal Cerebral Ischemia and Electrocardiology – Ph.D. thesis in Mthematical Engineeing – MOX, Dipartimento di Matematica, Politecnico di Milano – 2010 -Advisor: A. Veneziani
5. E. Schenone – Reduced models in cardiac electrophysiology Applications to the inverse problem – MSc in Mathematical Engineering – Politecnico di Milano – Advisor: L. Formaggia – A.A ’10-’11
6. S. Savare’ – Studio computazionale di ottimizzazione della stimolazione nella terapia di risincronizzazione cardiaca, BSc in Mathematical Engineering, Politecnico di Milano – Advisor: L. Formaggia and C. Vergara – A.A. ’13-’14.