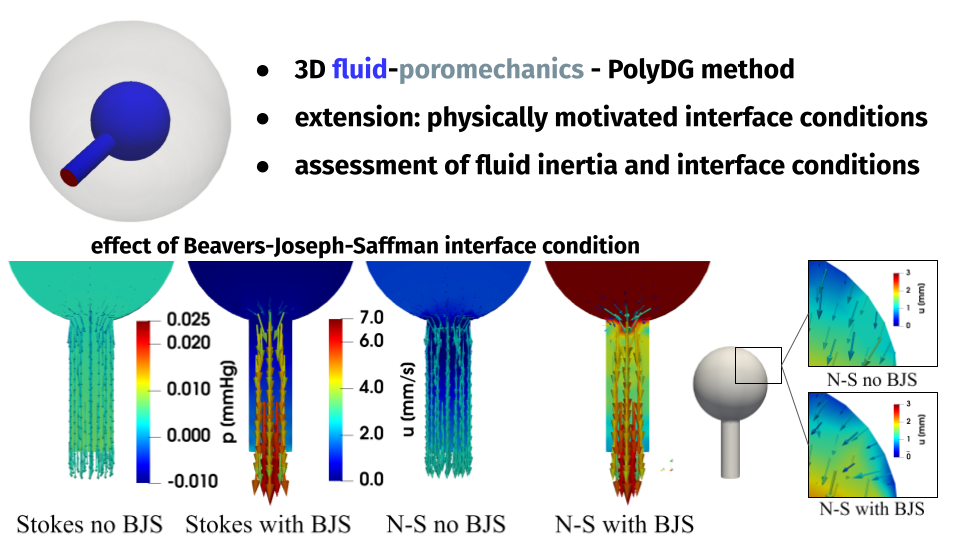
A new MOX Report entitled “Discontinuous Galerkin method for a three-dimensional coupled fluid-poroelastic model with applications to brain fluid mechanics” by Fumagalli, I. has appeared in the MOX Report Collection. Check it out here: https://www.mate.polimi.it/biblioteca/add/qmox/44-2024.pdf Abstract: The modeling of the interaction between a poroelastic medium and a fluid in a hollow cavity is crucial for understanding, e.g., the multiphysics flow of blood and Cerebrospinal Fluid (CSF) in the brain, the supply of blood by the coronary arteries in heart perfusion, or the interaction between groundwater and rivers or lakes. In particular, the cerebral tissue’s elasticity and its perfusion by blood and interstitial CSF can be described by Multi-compartment Poroelasticity (MPE), while CSF flow in the brain ventricles can be modeled by the (Navier-)Stokes equations, the overall system resulting in a coupled MPE-(Navier-)Stokes system. The aim of this paper is three-fold. First, we aim to extend and verify in a three-dimensional setting a discontinuous Galerkin method on polytopal grids recently presented for the MPE-Stokes problem. Second, we carry out the analysis of the method based on an extension of the proposed formulation so that physics-bas! ed Beaver s-Joseph-Saffman conditions are taken into account at the interface: these conditions are essential to model the friction between the fluid and the porous medium. Finally, by a comparative numerical investigation, we assess the fluid-dynamics effects of these boundary conditions and of employing either Stokes or Navier-Stokes equations to model the CSF flow. The semidiscrete numerical scheme for the coupled problem is proved to be stable and optimally convergent. Temporal discretization is obtained using Newmark’s beta-method for the elastic wave equation and the theta-method for the remaining equations of the model. The theoretical error estimates are verified by numerical simulations on a test case with a manufactured solution, and a numerical investigation is carried out on a three-dimensional geometry to assess the effects of interface conditions and fluid inertia on the system.
