Sedimentary basins are among the best places to find petroleum and natural gas and to store nuclear waste material. The history of the basin has a deep impact on the characteristic of the oil generated: the evolution and the temperature of the sediments determine localization, quantity and quality of the oil. Moreover, oil usually floats and collects near cap-Rock. Thus, to have a detailed information about oil, we need the information on the past of the basin.
Geologists can outline different scenarios of the basin and they have to choose among them the one that is coherent from the physical viewpoint. A numerical simulation could be very effective tool to make this decision, in fact it can provide quantitative information about the physics of the basin, e.g. stress and temperature field. Moreover this approach does not have the same technical difficulties and large economic impact that is carried out when we are dealing with analogical experiments.
Now the issue becomes: how can we make a good numerical simulation? In this new framework there are various difficulties to be faced. One of the main issue is the definition of the geometrical domain. In fact numerical simulation are usually based on the resolution of Partial Differential Equations defined on a discrete domain and the result of these equations is effected by a “good” approximation of such domain.
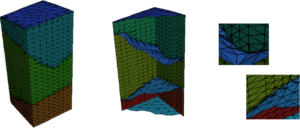
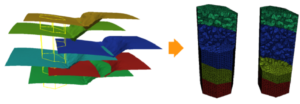
Data from seismic imaging provide a description of the horizons, i.e., bedding surfaces where there is a marked change in the composition of rocks. To proceed with a numerical simulation, we are often demanded to furnish a volume discretization, i.e., a volume composed by tetrahedral elements, representing the whole sedimentary basin, moving from these discrete surfaces.

Consequently, to get a proper computational domain for a numerical simulation we need a lot of basic operation:
[one_third]
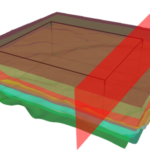
[/one_third][two_third_last]surface intersection;[/two_third_last]
[one_third]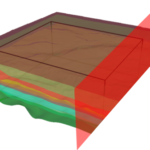 [/one_third][two_third_last]surface cutting;[/two_third_last]
[/one_third][two_third_last]surface cutting;[/two_third_last]
[one_third] [/one_third][two_third_last]shape improvement;[/two_third_last]
[/one_third][two_third_last]shape improvement;[/two_third_last]
[one_third]
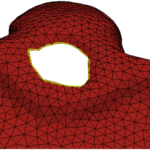 [/one_third][two_third_last]fill any lack of data.[/two_third_last]
[/one_third][two_third_last]fill any lack of data.[/two_third_last]
The shape of the tetrahedral elements can decrease the accuracy and the performance of a numerical method and, in the worst cases, only one badly-shaped element is able to “block” the process and derail the numerical simulation. These badly-shaped elements are the tetrahedrons characterized by small volumes and large dihedral angles.
Examples of basins