Geometrical multiscale

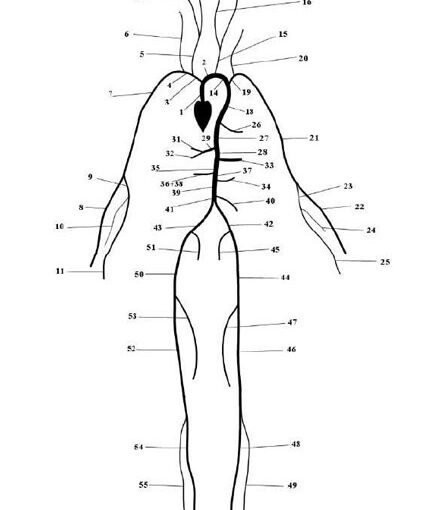
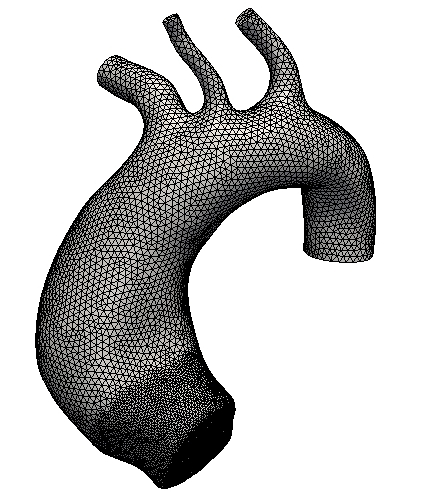
The geometrical multiscale approach consists in the coupling of spatial heterogeneous models for the description of the cardiovascular system. Usually, one considers 3D models (either in compliant or rigid vessels) to describe the portion of the system where a detailed description is needed (e.g., the ascending aorta, the carotids, …), 1D models for the description of the main arteries and veins to simulate the pressure wave propagation, and 0D models for the description of the peripheral vessels and capillaries.

The coupling among these different models is obtained by introducing suitable interface conditions involving e.g. the pressure or the mean pressure, the velocity or the flow rate, the characteristic variables, the total pressure.

MOX Laboratory has been one of the first center worldwide that introduced and studied this approach. The research focused on the well-posedness of the continuous coupled problem, on the development and analysis of suitable numerical strategies for its solution, and on the application to cases of clinical interest.

Strictly related to the geometrical multiscale approach is the treatment of the so-called defective boundary conditions arising when a 1D or 0D model is coupled with a 3D model. Indeed, in these cases, the reduced models are not able to provide all the information required by the 3D model to be well-posed. Several strategies were proposed and analyzed for the prescription of flow rate, mean pressure and resistance conditions.

Publications:

1. L. Formaggia, F. Nobile, A. Quarteroni, and A. Veneziani. Multiscale modelling of the circulatory system: a preliminary analysis. Comput. Vis. Sci., 2(2-3), pp. 75–83, 1999
2. A. Quarteroni, M. Tuveri and A. Veneziani. Computational vascular fluid dynamics: problems, models and methods, Computing and Visualization in Science, 2(4), pp. 163-97, 2000.
3. L. Formaggia, J.-F. Gerbeau, F. Nobile, and A. Quarteroni, On the coupling of 3D an 1D Navier-Stokes equations for flow problems in compliant vessels. Comput. Methods Appl. Mech. Engrg., 191(6-7), pp. 561–582, 2001
4. A. Quarteroni, S. Ragni, A. Veneziani, Coupled between lumped and distributed models for blood flow problems, Comp Vis Science, 4(2), pp.111-124, 2001
5. K. Lagan`, G. Dubini, F. Migliavacca, R. Pietrabissa, A. Quarteroni, S. Ragni, A. Veneziani, Multiscale Modeling as a a tool to prescribe realistic boundary conditions for the study of surgical procedures, Biorheology, 39 pp. 359-364, 2002
6. L. Formaggia, J.-F. Gerbeau, F. Nobile, and A. Quarteroni. Numerical treatment of defective boundary conditions for the Navier-Stokes equation. SIAM J. Numer. Anal., 40(1), pp. 376–401, 2002
7. A. Quarteroni and A. Veneziani. Analysis of a geometrical multiscale model based on the coupling of ODEs and PDEs for blood flow simulations, Multiscale Modeling and Simulation, 1(2), pp. 173-195, 2003
8. V. Milisic and A. Quarteroni. Analysis of lumped parameter models for blood flow simulations and their relation with 1D models, Mathematical Modelling and Numerical Analysis, 38(4), pp. 613-32, 2004
9. Veneziani A., Vergara C., Flow rate defective Boundary Conditions in Haemodynamics Simulations, Int. Journ. Num. Meth. Fluids, 47, pp. 803–816, 2005
10. M. A. Fernández, V. Milisic and A. Quarteroni. Analysis of a geometrical multiscale blood flow model based on the coupling of ODEs and hyperbolic PDEs, Multiscale Modeling and Simulation, 4(1), pp. 215-236, 2005
11. L. Formaggia, D. Lamponi, A. Veneziani, and M. Tuveri. Numerical modeling of 1D arterial networks coupled with a lumped parameters description of the heart. Computer Methods in Biomechanics and Biomedical Engineering, 9(5), pp. 273–288, 2006
12. L. Formaggia, A. Moura, and F. Nobile. On the stability of the coupling of 3D and 1D
    fluid-structure interaction models for blood flow simulations. Mathematical modelling and numerical analysis, 41(4), pp. 743–769, 2007
13. Veneziani A., Vergara C., An approximate method for solving incompressible Navier-Stokes problem with flow rate conditions, Comp. Meth. Appl. Mech. Eng., 196 (9-12), pp. 1685-1700, 2007
14. C. D’Angelo and A. Quarteroni. On the coupling of 1D and 3D Diffusion-Reaction Equations: Application to Tissue Pefusion Problems, Mathematical Models and Methods in Applied Sciences, 18(8), pp. 1481 – 1504, 2008
15. Formaggia L., Veneziani A., Vergara C., A new approach to numerical solution of defective boundary value problems in incompressible fluid dynamics, SIAM J. Num. Anal., 46(6), pp. 2769-2794, 2008
16. P. Zunino, Numerical approximation of incompressible ows with net ux defective boundary conditions by means of penalty techniques, Comput. Methods Appl. Mech. Engrg., 198(37-40), pp. 3026-3038, 2009
17. T. Passerini, M. R. De Luca, L. Formaggia, A. Quarteroni and A. Veneziani. A 3D/1D geometrical Multiscale Model of the Cerebral Vasculature, Journal of Engineering Mathematics, 2009
18. R. Balossino, G. Pennati, F. Migliavacca, L. Formaggia, A. Veneziani, M. Tuveri, and G. Dubini. Computational models to predict stenosis growth in carotid arteries: Which is the role of boundary conditions? Comp. Meth. Biomech. Biomed. Engng., 12(1), pp. 113–123, 2009
19. Formaggia L., Veneziani A., Vergara C., Flow rate boundary problems for an incompressible fluid in deformable domains: formulations and solution methods. Comp. Meth. Appl. Mech. Eng., 199 (9-12), pp. 677-688, 2010
20. A. C. I. Malossi, P. J. Blanco, S. Deparis and A. Quarteroni. Algorithms for the partitioned solution of weakly coupled fluid models for cardiovascular flows, International Journal for Numerical Methods in Biomedical Engineering, 27(12), pp. 2035-2057, 2011
21. Vergara C., Nitsche’s method for defective boundary value problems in incompressibile fluid-dynamics. Journal of Scientific Computing, 46, pp. 100-123, 2011
22. P. J. Blanco, M. Discacciati and A. Quarteroni. Modeling dimensionally-heterogeneous problems: analysis, approximation and applications, Numerische Mathematik, 119, pp. 299-335, 2011
23. M. Lesinigo, C. D’Angelo and A. Quarteroni. A multiscale Darcy-Brinkman model for fluid flow in fractured porous media, Numerische Mathematik, 117, pp. 717-752, 2011
24. Porpora A., Zunino P., Vergara C., Piccinelli M., Numerical treatment of boundary conditions to replace lateral branches in haemodynamics. Int. J. Numer. Meth. Biomed. Eng., 28(12), pp. 1165-1183, 2012
25. Formaggia L. and Vergara C., Prescription of general defective boundary conditions in fluid-dynamics. Milan Journal of Mathematics, 80(2), pp. 333-350, 2012
26. A. C. I. Malossi, P. J. Blanco, P. Crosetto, S. Deparis and A. Quarteroni. Implicit coupling of one-dimensional and three-dimensional blood flow models with compliant vessels, Multiscale Modeling and Simulation, 11(2), pp. 474-506, 2013
27. L. Formaggia, A. Quarteroni and C. Vergara. On the physical consistency between three-dimensional and one-dimensional models in haemodynamics, Journal Of Computational Physics, 244, pp. 97-112, 2013
28. P. Reymond, P. Crosetto, S. Deparis, A. Quarteroni and N. Stergiopulos. Physiological simulation of blood flow in the aorta: comparison of hemodynamic indices as predicted by 3-D FSI, 3-D rigid wall and 1-D models, Medical Engineering and Physics, vol. 35, num. 6, p. 784-791, 2013
29. Quarteroni A., Veneziani A., Vergara C., Geometric multiscale modeling of the cardiovascular system, between theory and practice. Comp. Meth. Appl. Mech. Eng., 302, pp. 193–252, 2016
30. Quarteroni A., Manzoni A., Vergara C., The Cardiovascular System: Mathematical Modeling, Numerical Algorithms, Clinical Applications. MOX Report n. 38/2016

Thesis

1. Passerini Tiziano, Modelli multiscala per il sistema circolatorio: accoppiamento numerico di modelli tridimensionali e monodimensionali, Biomedical Engineering, AA ’03-’04. Advisor: A. Veneziani
2. Invernizzi Simona, Analisi e simulazione numerica di problemi accoppiati monodimensionali-zerodimensionali in fluidodinamica, Aerospace Engineering, AA ’04-’05. Advisor: A. Quarteroni
3. Pischedda Marco, Condizioni al bordo realistiche per le equazioni di Navier-Stokes in emodinamica cerebrale, Mathematical Engineering, AA ’05-’06. Advisor: A. Veneziani
4. Vergara Christian, Numerical Modeling of Defective Boundary Problems in Incompressible Fluid-Dynamics, PhD thesis, 2006. Advisor: A. Veneziani
5. Moura Alexandra, The Geometrical Multiscale Modelling of the Cardiovascular System: Coupling 3D FSI and 1D Models, PhD thesis, 2007. Advisors: L. Formaggia, F. Nobile
6. Mariani Pietro, Implementazione ad oggetti di modelli a parametri concentrati del sistema circolatorio, MSc in Mathematical Engineering, AA ’07-’08. Advisor: L. Formaggia
7. Passerini Tiziano, Computational hemodynamics of the cerebral circulation: multiscale modeling from the circle of Willis to cerebral aneurysms, PhD thesis, 2009. Advisor: A. Veneziani
8. G. Scuderi – Numerical study of the coupling between heterogeneous models in computational haemodynamics – MSc in Mathematics – Universita’ di Milano Bicocca – Academic year ’10-’11 – Advisors: A. Russo and L. Formaggia

Links

– One dimensional modeling of the arterial and venous network

– Blood flow in arteries and veins