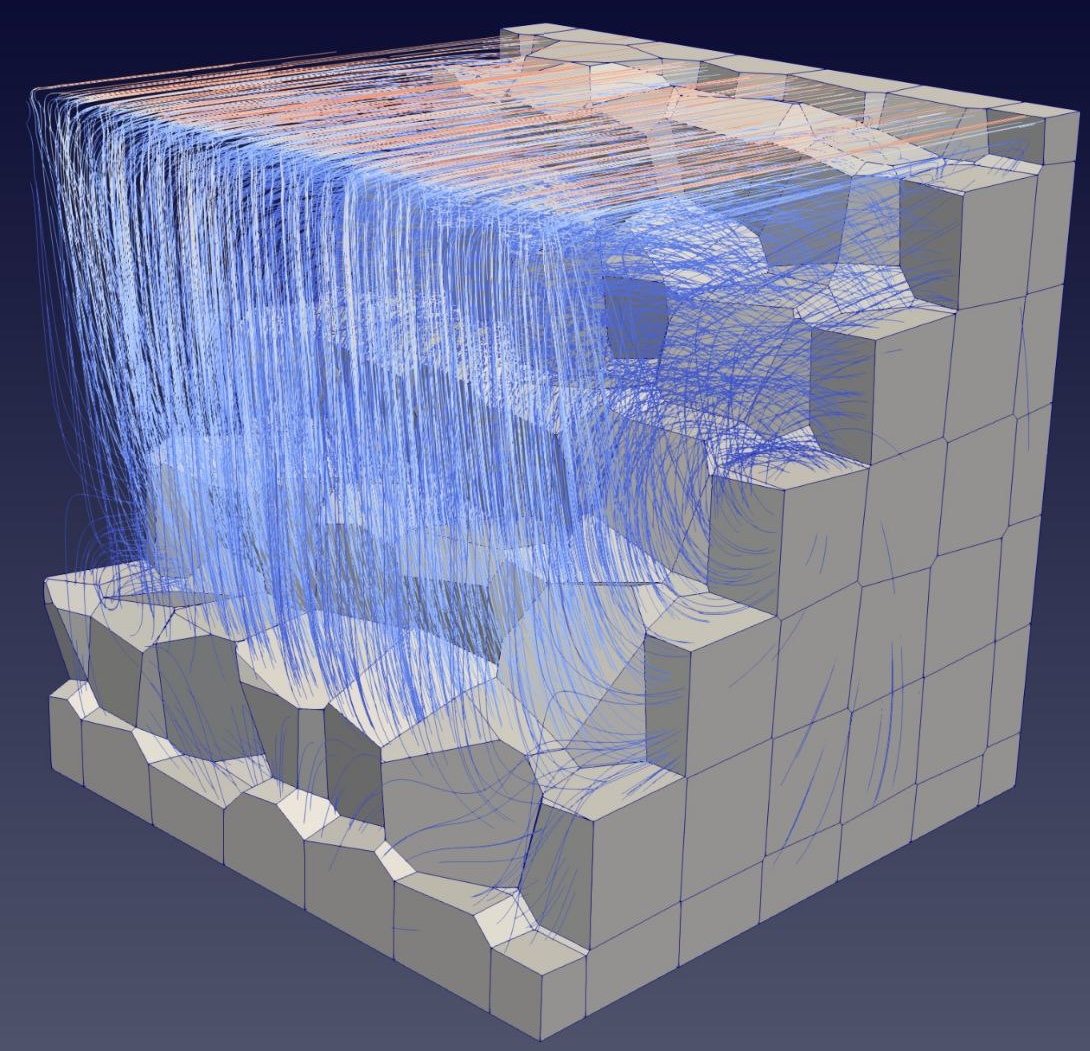
A new MOX Report entitled “Stability, convergence, and pressure-robustness of numerical schemes for incompressible flows with hybrid velocity and pressure” by Botti, L.; Botti, M.; Di Pietro, D.A.; Massa; F.C. has appeared in the MOX Report Collection. Check it out here: https://www.mate.polimi.it/biblioteca/add/qmox/35-2024.pdf Abstract: In this work we study the stability, convergence, and pressure-robustness of discretization methods for incompressible flows with hybrid velocity and pressure. Specifically, focusing on the Stokes problem, we identify a set of assumptions that yield inf-sup stability as well as error estimates which distinguish the velocity- and pressure-related contributions to the error. We additionally identify the key properties under which the pressure-related contributions vanish in the estimate of the velocity, thus leading to pressure-robustness. Several examples of existing and new schemes that fit into the framework are provided, and extensive numerical validation of the theoretical properties is provided.
You may also like
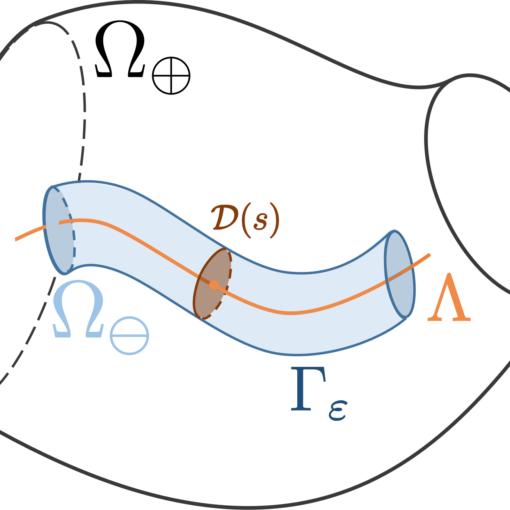
A new MOX Report entitled “Robust Preconditioning of Mixed-Dimensional PDEs on 3d-1d domains coupled with Lagrange Multipliers” by Dimola N.; Kuchta M.; […]
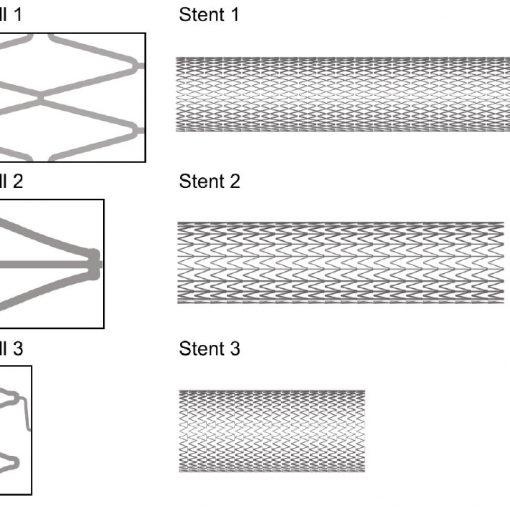
A new MOX Report entitled “Easy-to-use formulations based on the homogenization theory for vascular stent design and mechanical characterization” by Carbonaro, D.; […]
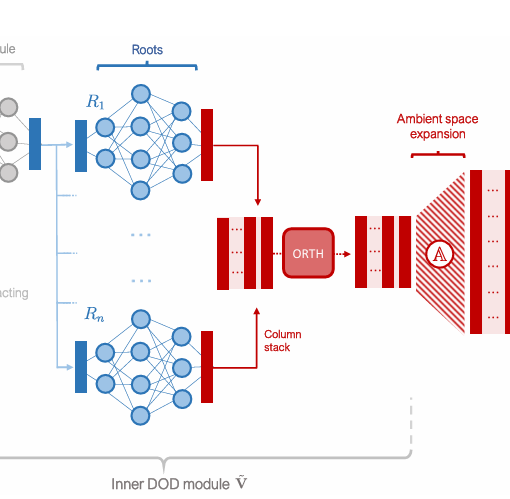
A new MOX Report entitled “Deep orthogonal decomposition: a continuously adaptive neural network approach to model order reduction of parametrized partial differential […]
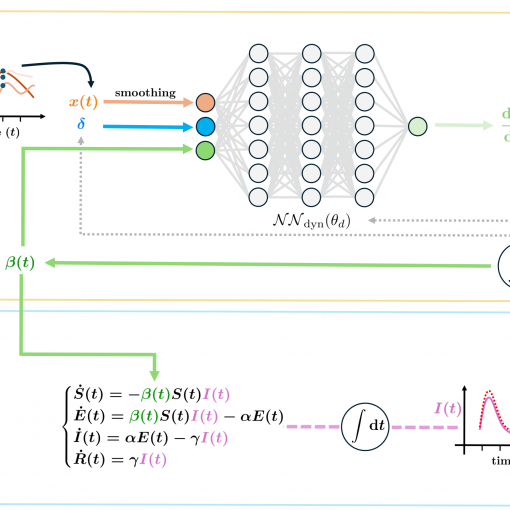
A new MOX Report entitled “A model learning framework for inferring the dynamics of transmission rate depending on exogenous variables for epidemic […]