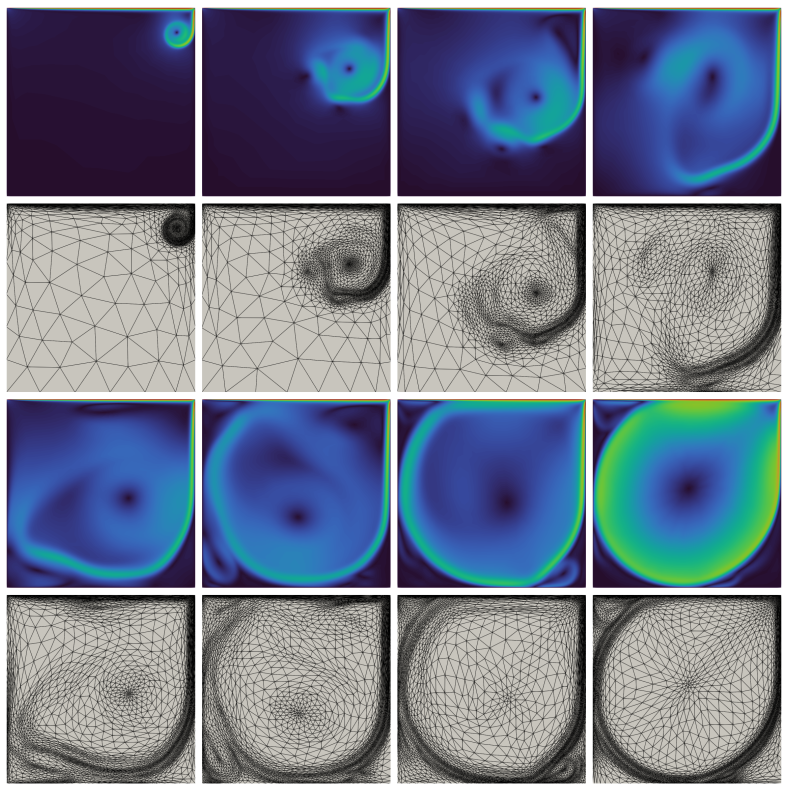
A new MOX Report entitled “Space – time mesh adaptation for the VMS – Smagorinsky modeling of high Reynolds number flows ” by Temellini, E.; Ferro, N.; Stabile, G.; Delgado Avila, E.; Chacon Rebollo, T.; Perotto, S. has appeared in the MOX Report Collection. Check it out here: https://www.mate.polimi.it/biblioteca/add/qmox/60-2024.pdf Abstract: Traditional methods, such as Reynolds-Averaged Navier-Stokes (RANS) equations and Large Eddy Simulations (LES), provide consolidated tools for the numerical approximation of high Reynolds number flows in a wide range of applications – from green energy to industrial design. In general, RANS modeling is practical when the main interest is the time-averaged flow behavior. LES equations offer detailed insights into flow dynamics and a more accurate solution, but the high computational demand necessitates innovative strategies to reduce costs while maintaining precision. In this study, we enhance the Variational MultiScale (VMS)-Smagorinsky LES model by relying on an adaptive discretization strategy in both space and time, driven by a recovery-based a posteriori error analysis. We assess the effectiveness of the approach in capturing flow characteristics across a wide range of Reynolds numbers through benchmark tests.
You may also like
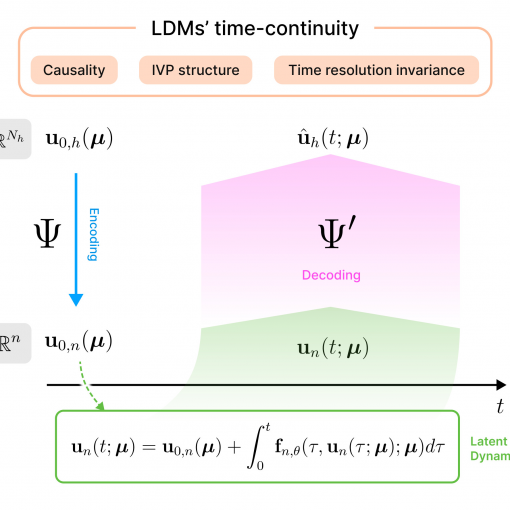
A new MOX Report entitled “On latent dynamics learning in nonlinear reduced order modeling” by Farenga, N.; Fresca, S.; Brivio, S.; Manzoni, […]
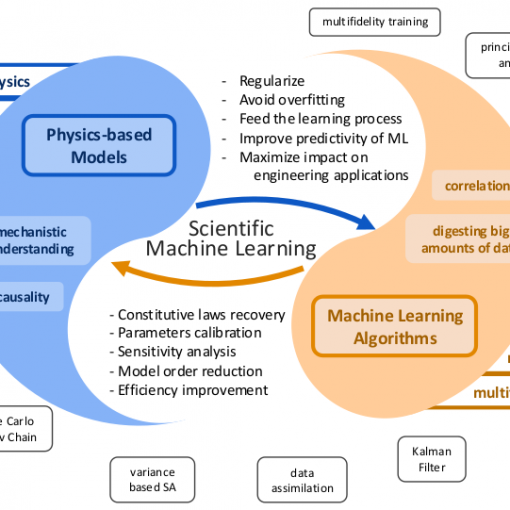
A new MOX Report entitled “Combining physics-based and data-driven models: advancing the frontiers of research with Scientific Machine Learning” by Quarteroni, A.; […]
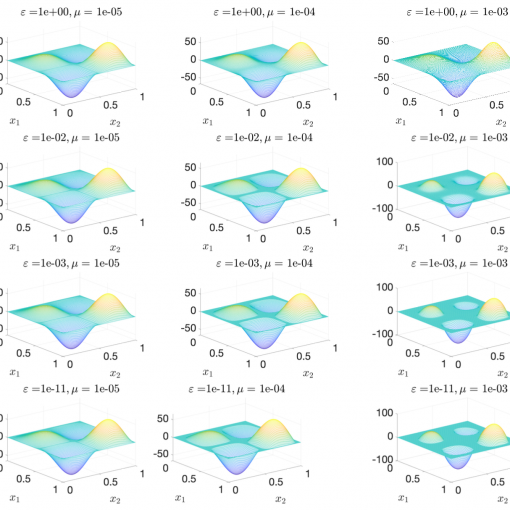
A new MOX Report entitled “Solving Semi-Linear Elliptic Optimal Control Problems with L1-Cost via Regularization and RAS-Preconditioned Newton Methods” by Ciaramella, G.; […]
A new MOX Report entitled “Unified discontinuous Galerkin analysis of a thermo/poro-viscoelasticity model” by Bonetti, S.; Corti, M. has appeared in the […]