A new MOX report entitled “On the evolution equations of interfacial variables in two-phase flows” by Orlando, G; Barbante, P.F.; Bonaventura, L. has appeared in the MOX Report Collection.
The report can be donwloaded at the following link:
https://www.mate.polimi.it/biblioteca/add/qmox/55-2023.pdf
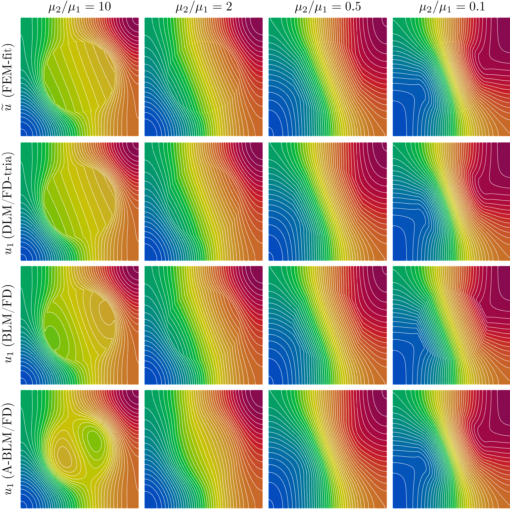
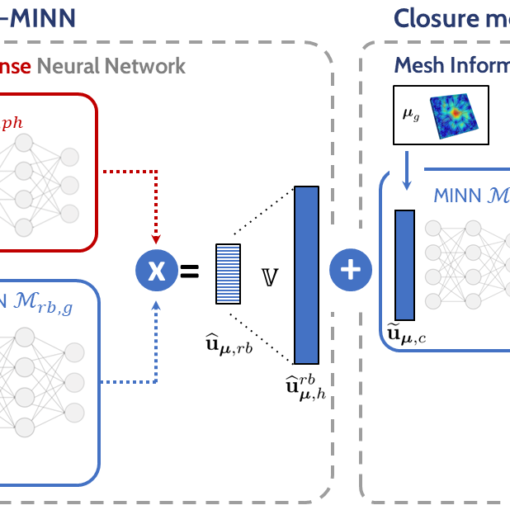
Abstract: Many physical situations are characterized by interfaces with a non trivial shape so that relevant geometric features, such as interfacial area, curvature or unit normal vector, can be used as main indicators of the topology of the interface. We analyze the evolution equations for a set of geometrical quantities that characterize the interface in two-phase flows. Several analytical relations for the interfacial area density are reviewed and presented, clarifying the physical significance of the different quantities involved and specifying the hypotheses under which each transport equation is valid. Moreover, evolution equations for the unit normal vector and for the curvature are analyzed. The impact of different formulations is then assessed in numerical simulations of rising bubble benchmarks.