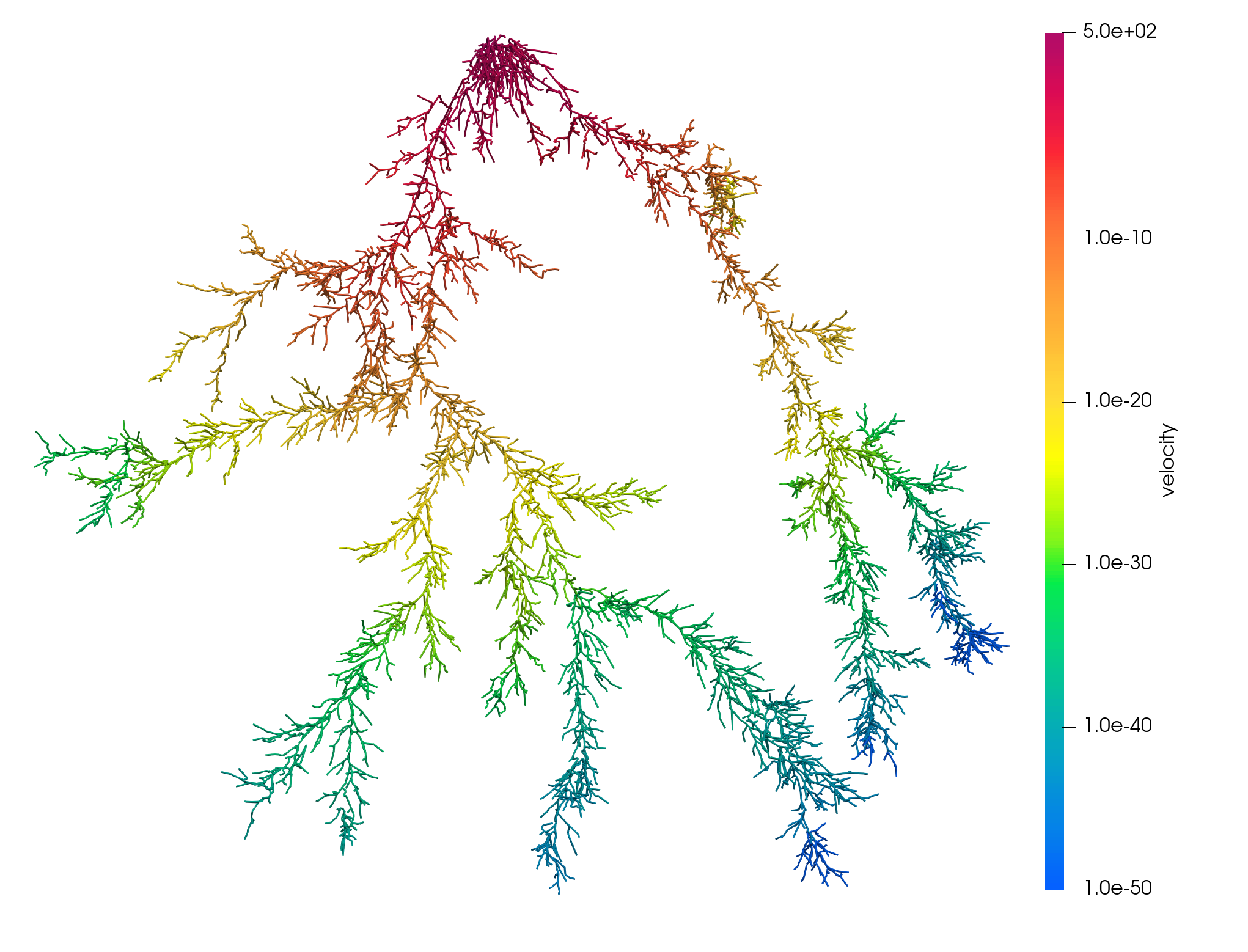
A new MOX Report entitled “Numerical Solution of linear drift-diffusion and pure drift equations on one-dimensional graphs” by Crippa, B.; Scotti, A.; Villa, A has appeared in the MOX Report Collection. Check it out here: https://www.mate.polimi.it/biblioteca/add/qmox/80-2024.pdf Abstract: We propose numerical schemes for the approximate solution of problems defined on the edges of a one-dimensional graph. In particular, we consider linear transport and a drift-diffusion equations, and discretize them by extending Finite Volume schemes with upwind flux to domains presenting bifurcation nodes with an arbitrary number of incoming and outgoing edges, and implicit time discretization. We show that the discrete problems admit positive unique solutions, and we test the methods on the intricate geometry of an electrical treeing.
You may also like
A new MOX Report entitled “Modelling of initially stressed solids: structure of the energy density in the incompressible limit” by Magri, M.; […]
A new MOX Report entitled “A scalable well-balanced numerical scheme for the modelling of two-phase shallow granular landslide consolidation” by Gatti, F.; […]
A new MOX Report entitled “Multi-fidelity surrogate modeling using long short-term memory networks” by Conti, P.; Guo, M.; Manzoni, A.; Hesthaven, J.S. […]
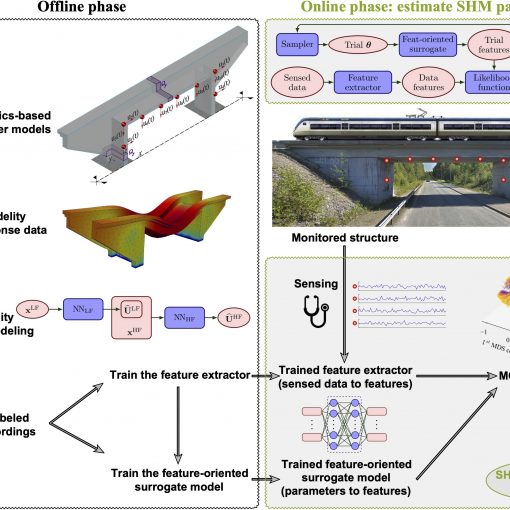
A new MOX Report entitled “Enhancing Bayesian model updating in structural health monitoring via learnable mappings” by Torzoni, M.; Manzoni, A.; Mariani, […]