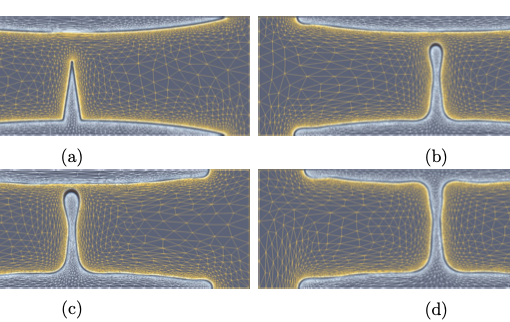
A new MOX Report entitled “A Necas-Lions inequality with symmetric gradients on star-shaped domains based on a first order Babuska-Aziz inequality” by Botti, M.; Mascotto, L. has appeared in the MOX Report Collection. Check it out here: https://www.mate.polimi.it/biblioteca/add/qmox/52-2024.pdf Abstract: We prove a Necas-Lions inequality with symmetric gradients on two and three dimensional domains that are star-shaped with respect to a ball B; the constants in the inequality are explicit with respect to the diameter and the radius of B. Crucial tools in deriving this inequality are a first order Babuska-Aziz inequality based on Bogovskii’s construction of a right-inverse of the divergence and Fourier transform techniques proposed by Duran. As a byproduct, we derive arbitrary order estimates in arbitrary dimension for that operator.
You may also like
A new MOX Report entitled “Structure-preserving neural networks in data-driven rheological models” by Parolini, N.; Poiatti, A.; Vene’, J.; Verani, M. has […]
A new MOX Report entitled “Modeling and simulation of electrochemical and surface diffusion effects in filamentary cation-based resistive memory devices” by Vaccaro, […]
A new MOX Report entitled “Elucidating the cellular determinants of the end-systolic pressure-volume relationship of the heart via computational modelling” by Regazzoni, […]
A new MOX Report entitled ” Estimation of dynamic Origin–Destination matrices in a railway transportation network integrating ticket sales and passenger count […]