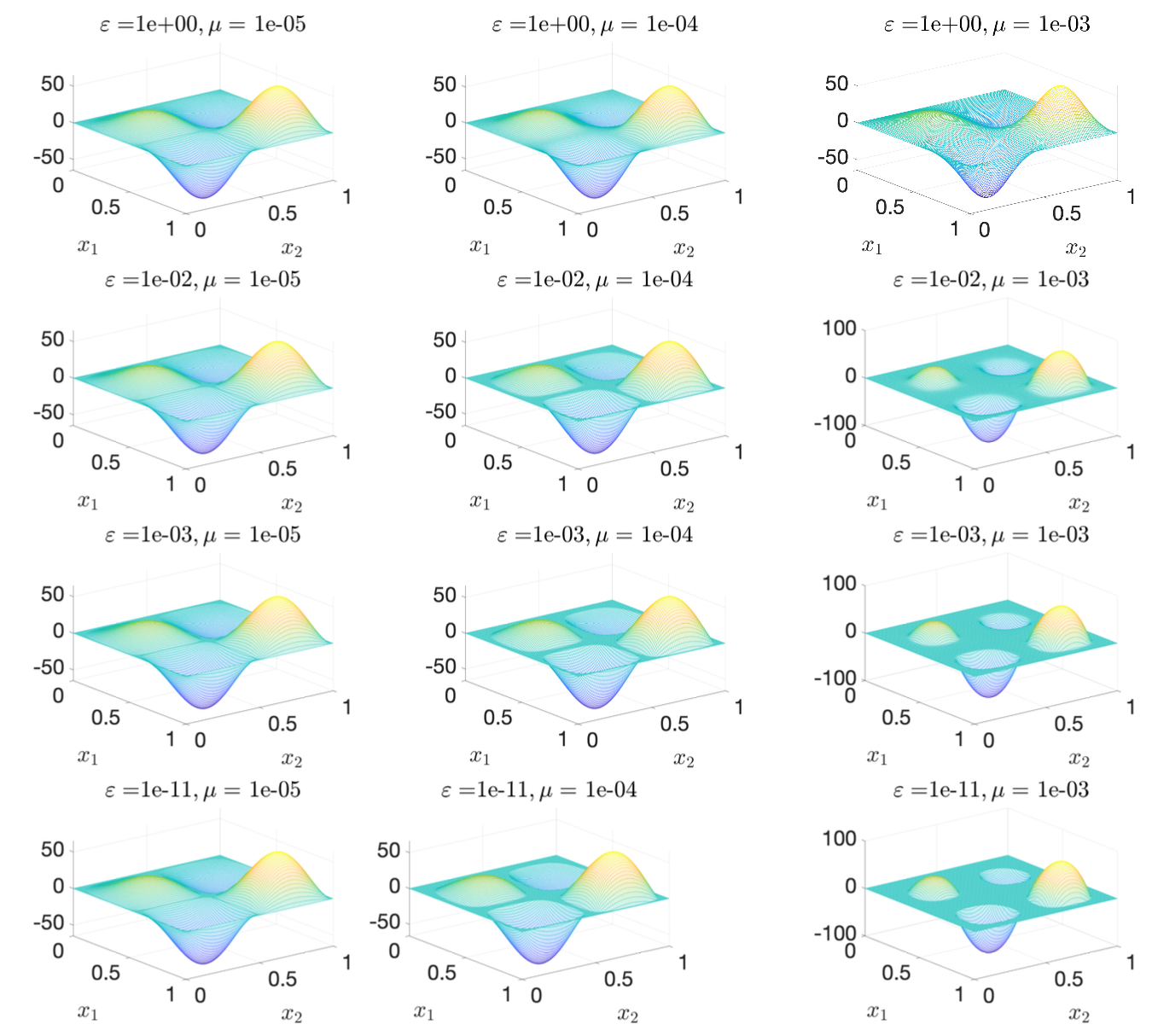
A new MOX Report entitled “Solving Semi-Linear Elliptic Optimal Control Problems with L1-Cost via Regularization and RAS-Preconditioned Newton Methods” by Ciaramella, G.; Kartmann, M.; Mueller, G. has appeared in the MOX Report Collection. Check it out here: https://www.mate.polimi.it/biblioteca/add/qmox/91-2024.pdf Abstract: We present a new parallel computational framework for the efficient solution of a class of L2/L1-regularized optimal control problems governed by semi-linear elliptic partial differential equations (PDEs). The main difficulty in solving this type of problem is the nonlinearity and non-smoothness of the L1-term in the cost functional, which we address by employing a combination of several tools. First, we approximate the non-differentiable projection operator appearing in the optimality system by an appropriately chosen regularized operator and establish convergence of the resulting system solutions. Second, we apply a continuation strategy to control the regularization parameter to improve the behavior of (damped) Newton methods. Third, we combine Newton’s method with a domain-decomposition-based nonlinear preconditioning, which improves its robustness properties and allows for parallelization. The efficiency of the proposed numerical framework! is demon strated by extensive numerical experiments.
You may also like
A new MOX Report entitled “Nonparametric tests for semiparametric regression models” by Ferraccioli, F.; Sangalli, L.M.; Finos, L. has appeared in the […]
A new MOX Report entitled “A gradient flow approach for combined layout-control design of wave energy parks” by Gambarini, M.; Ciaramella, G.; […]
A new MOX Report entitled “Stability, convergence, and pressure-robustness of numerical schemes for incompressible flows with hybrid velocity and pressure” by Botti, […]
A new MOX Report entitled “Structure-preserving neural networks in data-driven rheological models” by Parolini, N.; Poiatti, A.; Vene’, J.; Verani, M. has […]