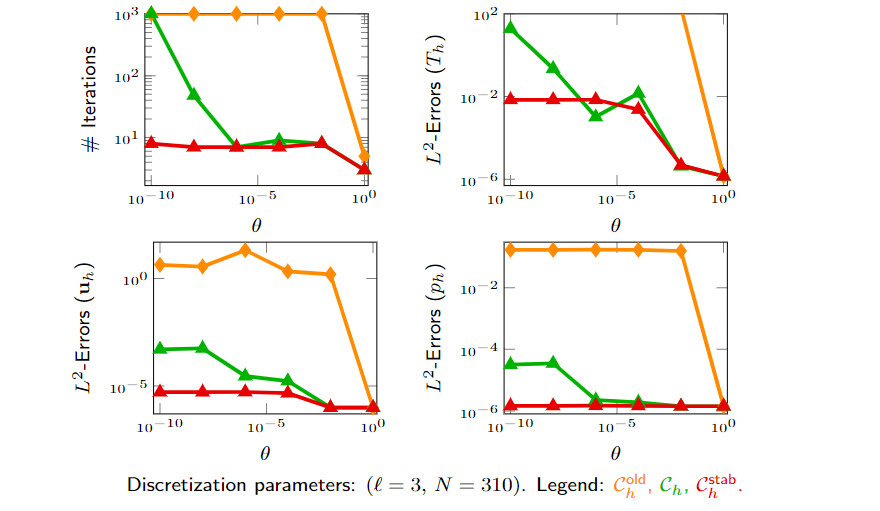
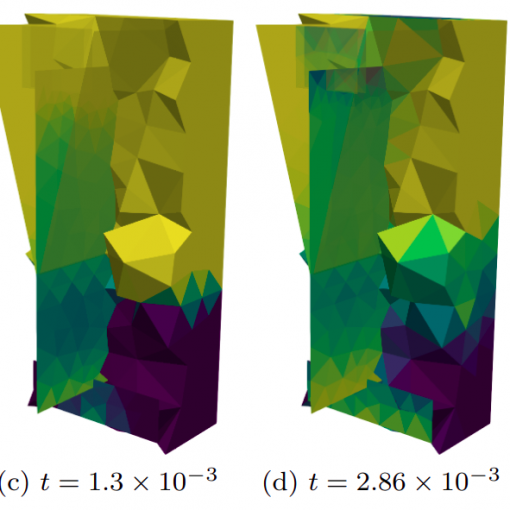
A new MOX Report entitled “Robust discontinuous Galerkin-based scheme for the fully-coupled non-linear thermo-hydro-mechanical problem” by Bonetti, S.; Botti, M.; Antonietti, P.F. has appeared in the MOX Report Collection.
Check it out here: https://www.mate.polimi.it/biblioteca/add/qmox/96-2023.pdf
Abstract: We present and analyze a discontinuous Galerkin method for the numerical modeling of the non-linear fully-coupled thermo-hydro-mechanic problem. We propose a high-order symmetric weighted interior penalty scheme that supports general polytopal grids and is robust with respect to strong heteorgeneities in the model coefficients. We focus on the treatment of the non-linear convective transport term in the energy conservation equation and we propose suitable stabilization techniques that make the scheme robust for advection-dominated regimes. The stability analysis of the problem and the convergence of the fixed-point linearization strategy are addressed theoretically under mild requirements on the problem’s data. A complete set of numerical simulations is presented in order to assess the convergence and robustness properties of the proposed method.