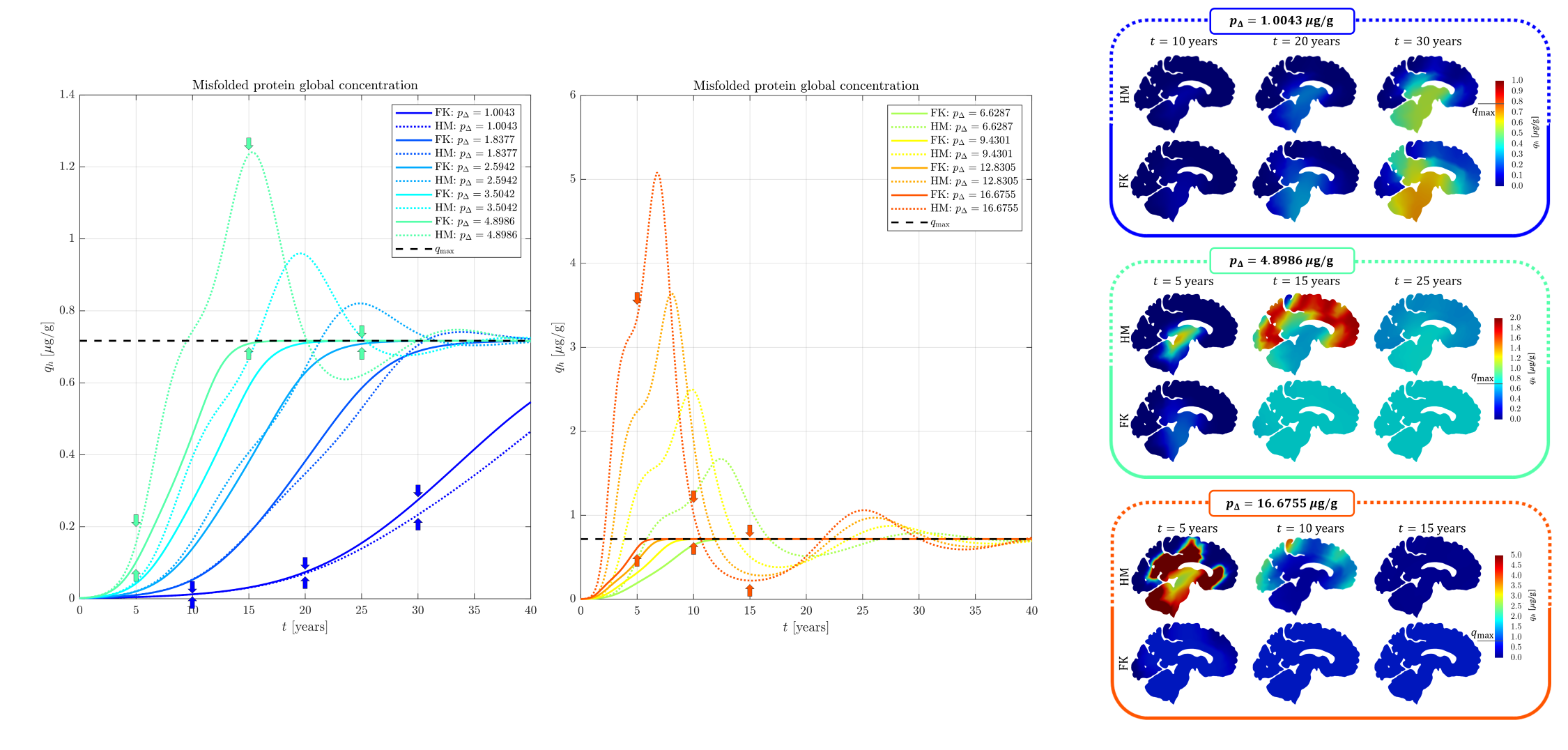
A new MOX Report entitled “Exploring tau protein and amyloid-beta propagation: a sensitivity analysis of mathematical models based on biological data” by Corti, M. has appeared in the MOX Report Collection. Check it out here: https://www.mate.polimi.it/biblioteca/add/qmox/34-2024.pdf Abstract: Alzheimer’s disease is the most common dementia worldwide. Its pathological development is well known to be connected with the accumulation of two toxic proteins: tau protein and amyloid-beta. Mathematical models and numerical simulations can predict the spreading patterns of misfolded proteins in this context. However, the calibration of the model parameters plays a crucial role in the final solution. In this work, we perform a sensitivity analysis of heterodimer and Fisher-Kolmogorov models to evaluate the impact of the equilibrium values of protein concentration on the solution patterns. We adopt advanced numerical methods such as the IMEX-DG method to accurately describe the propagating fronts in the propagation phenomena in a polygonal mesh of sagittal patient-specific brain geometry derived from magnetic resonance images. We calibrate the model parameters using biological measurements in the brain cortex for the tau protein and the amyloid-! beta in A lzheimer’s patients and controls. Finally, using the sensitivity analysis results, we discuss the applicability of both models in the correct simulation of the spreading of the two proteins.
